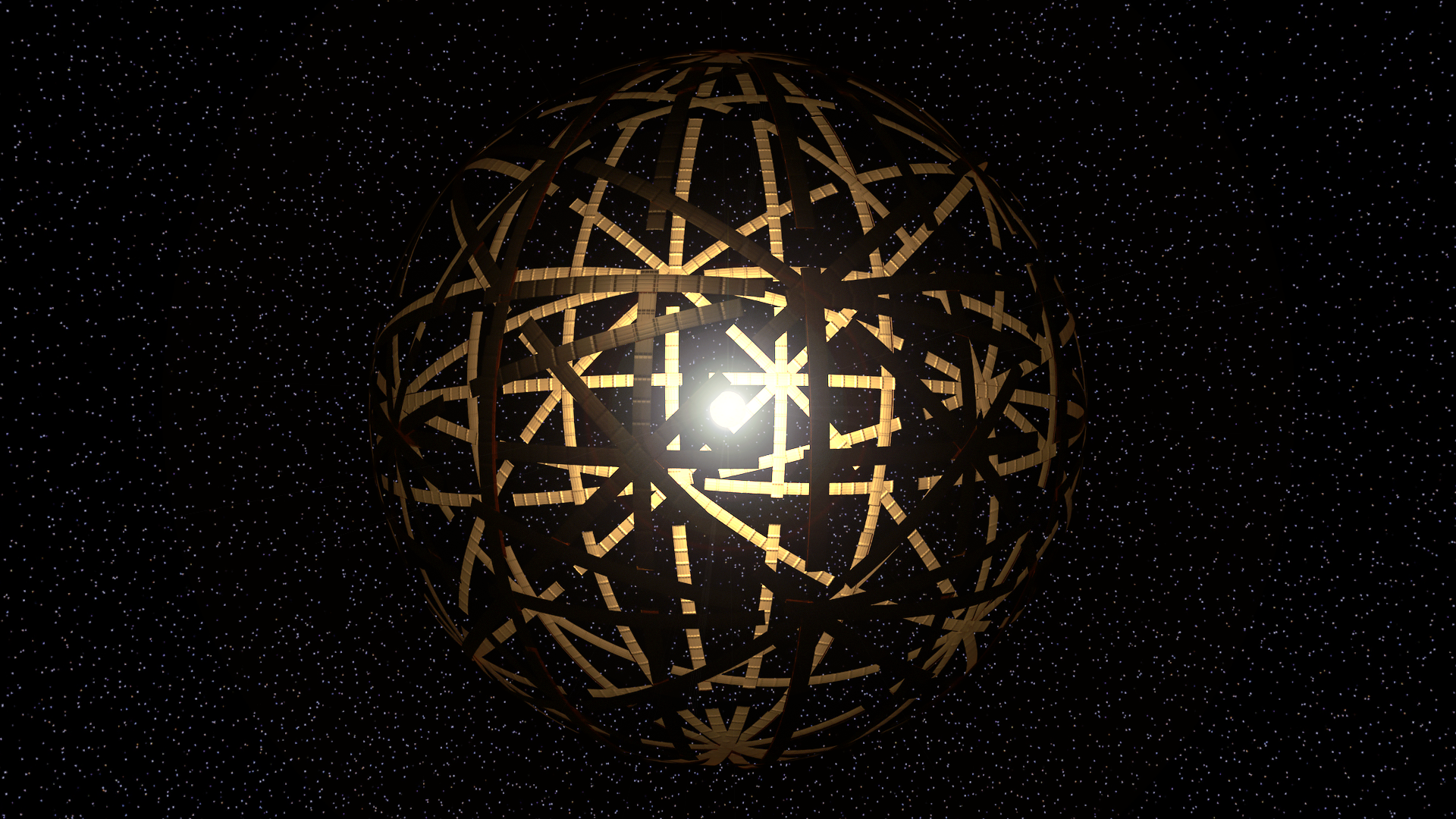
In 1960, visionary physicist Freeman Dyson proposed that an advanced alien civilization would someday quit fooling around with kindergarten-level stuff like wind turbines and nuclear reactors and finally go big, completely enclosing their home star to capture as much solar energy as they possibly could. They would then go on to use that enormous amount of energy to mine bitcoin, make funny videos on social media, delve into the deepest mysteries of the Universe, and enjoy the bounties of their energy-rich civilization.
But what if the alien civilization was… us? What if we decided to build a Dyson sphere around our sun? Could we do it? How much energy would it cost us to rearrange our solar system, and how long would it take to get our investment back? Before we put too much thought into whether humanity is capable of this amazing feat, even theoretically, we should decide if it’s worth the effort. Can we actually achieve a net gain in energy by building a Dyson sphere?
Spherical Dyson cows
I’ll state from the outset that I'm a theoretical cosmologist, not an engineer. I have absolutely no idea how to go about building a bridge, let alone a structure that reshapes the very face of our Solar System. But I’m willing to bet that nobody knows how to engage in these kinds of mega-engineering challenges. We can’t say for certain what kind of advances in which technologies would be necessary to build a structure that even partially encloses the sun. To speculate on that would be science fiction—fun, but not very meaty.
What I do know, though, is physics, and there are some things we can say about the physics of a Dyson sphere. We can use building one as a thought experiment to explore fundamental principles of energy, orbit, and motion. And this is important because no matter what technology-so-advanced-it’s-indistinguishable-from-magic our descendants come up with that allows them to rip apart planets, they still have to face the cold hard realities of our physics. They can’t get something for nothing. If they want to resculpt a planet, that takes energy. If they want to move a mountain-sized solar panel into a different orbit, that also takes energy.
For these and many other reasons, a Dyson sphere costs energy. So we’re going to see how long it will take to recoup the energy investment of building one and what the optimal design might be to minimize the initial investment.
To get at some numbers, we’re going to make a lot of assumptions. People like to poke fun at physicists for simplifying complex problems, sometimes beyond recognition. The old joke goes that dairy farmers reached out to a nearby university to help understand why milk production was low, and the response from the physicists began by assuming that the cows were spherical.
But there is something powerful about this simplifying approach, which is why physicists are trained in it from day one. First, it lets us answer questions when we’re not interested in precise numbers at the outset. Here, we just want a general sense of feasibility—will building a Dyson sphere take a (relatively) small, medium, or extreme amount of energy? Second, simplifying the problem helps cover up mistakes (either in calculations or our starting assumptions). If all we’re going after is a general ballpark, then a factor-of-two mistake (or even 10 or 100) won’t really change the overall intuitions our calculations enable.
Lastly, we literally don’t know how to build a Dyson sphere, so trying to go for anything more complex will simply lead to us introducing many more assumptions to handle all the small details. Each of those assumptions will increase the uncertainty of any numbers we produce, and that uncertainty will probably end up buried in the analysis rather than handily stated upfront.
Operating assumptions
As for the assumptions, here’s what I’ll do for the rest of this story. Feel free to make your own modifications—I sincerely hope this article provides not a formula for Dyson building but a springboard for fun discussion.
The goal here is to turn entire planets into solar energy harvesters. We don’t know and don’t care what method our descendants will use for energy capture and storage, so I’ll assume our energy harvester (e.g., a segment of the Dyson sphere) will be made of stuff that’s currently in rocks, so it will have the same average density as the Earth itself. I’ll keep this assumption when we move to dismantling other planets (focusing on their rocky portion as needed).
I’ll also assume that whatever elements we need to build our Dyson sphere will be present in the quantities we require. I figure this is a pretty fair assumption—after all, we’re talking about scooping up entire worlds and turning them into something else, so there’s a lot of material to work with.
Lastly, I’ll assume that our Dyson sphere will have a uniform thickness and density throughout its volume and that any segment of our sphere is a good enough approximation for its overall structure. It doesn’t matter if you go with the original Dyson sphere idea or just a “swarm” of gigantic panels. Either way, what I care about is the fraction of a sphere our structure will cover when placed at a particular orbit.
As for panel thickness and efficiency, we'll play with those numbers as we explore our options.
Unbind the Earth
Even if we were to coat the entire surface of the Earth in solar panels, we would still only capture less than a tenth of a billionth of all the energy our sun produces. Most of it just radiates uselessly into empty space. We’ll need to keep that energy from radiating away if we want to achieve Great Galactic Civilization status, so we need to do some slight remodeling. We don’t want just the surface of the Earth to capture solar energy; we want to spread the Earth out to capture more energy.
So we’re going to dismantle the Earth and turn it into giant, thin panels that orbit the sun, each one capturing light and turning it into energy. To get a general sense of the difficulty level here, we can turn to a quantity known as the binding energy. All the particles that make up the Earth are glued together by the force of their mutual gravitational attraction. If you want to disassemble the Earth, you could imagine picking one particle at a time and flinging it off at escape velocity.
This process gets easier as you go; with every particle gone, the gravity of the Earth reduces, making the escape velocity of the next particle a bit lower. Eventually, you’ll have removed every single particle from the planet and officially unbound our world. In fact, humans have already begun this process; we have successfully lofted approximately 10,000–20,000 metric tons of material into orbit and beyond (and a good fraction of it has even stayed there). We just have 5,971,999,999,999,999,990,000 metric tons to go and we’re golden.
While our descendants may concoct some ultra-clever way to minimize the effort needed to turn our planet into a series of flat panels, the binding energy gives us a good ballpark for the amount of energy required to do it. For the Earth, our binding energy is somewhere around 2.5x1032 Joules. To give you some perspective, every year, the entirety of humanity consumes around a mere 5x1020 Joules—a trillion times smaller.
Assuming we get the job of dismantling our planet done, it will be time to rearrange it into as much of a sphere as we can manage and then use that to start harnessing more solar energy than we can now. We’re ready to answer the key question: How long will it take to recoup the energy we spent in unbinding the Earth in the first place?
If we assume our shell has a thickness of 1 kilometer, that will give us a surface area equal to nearly 2,000 Earths. It won’t come close to covering our sun, however, as at our orbit, it could only capture around 0.0004 percent of all the sunlight. Still, that’s an enormous improvement from what we can get from a fully bound planet. Our sun blasts out about 3.8x1026 Joules of energy every single second. If we assume that our energy conversion process is 10 percent efficient, capturing even that tiny fraction allows us to recover our binding energy expenditure in only 60,000 years. Considering the scale of mega-engineering that we’re operating at, that’s not so bad.
If we can shrink the panel thickness to just one meter and increase the efficiency to 90 percent, we can pay back our energy investment in a handful of years. From then on, it’s just gravy.
What about other planets? If we’ve grown too fond of the Earth to take it to pieces, it’s not a problem—if we can do it here, we can do it anywhere. Mercury has the benefit of already being nice and close to the sun, so dismantling it will allow us to cover a larger fraction of the sun’s output. But it’s also a smaller world with less material to work with. With kilometer-thick panels made of Mercury (and not, you know, mercury), we could capture 0.0001 percent of the sun’s output. At 10 percent efficiency, we would recover our Mercury-unbinding cost in around a thousand years. With meter-thick panels and 90 percent efficiency, we would achieve a surface area equal to over 100,000 Earths and pay back our investment in less than a year.
At the other end of the spectrum, Jupiter is by far the most massive planet in the Solar System, so it should make for great Dyson-building. But it’s mostly gas; it only has about five Earth’s worth of rocky material (theoretically—we’re not sure) buried under thousands of kilometers of mostly useless gas. We'd have to unbind the whole dang thing, and then we don’t even get to use most of the mass of the planet. When all is said and done, we would get around 10,000 Earths' worth of surface area, but at that distant orbit, it’s no better than Mercury’s coverage ability. Given the enormous cost of unbinding that gas giant, it would take hundreds of millions of years to get our money back.
Switching to thinner panels and higher efficiency improves the situation somewhat, allowing us to get a positive ROI after only a few hundred thousand years. But we’re not an especially patient culture, so that would be a pretty tough sell.
Moving mountains
All these calculations assume that we leave each planet’s material in its present orbit. But if we’re going to engage in restructuring our Solar System, let’s go all the way. The amount of radiation we can capture with a given surface area decreases with the square of the distance from the sun. Shrink that distance and the energy goes up. If we could move our planet parts into a closer orbit, we could enclose a greater fraction of our star’s output.
But there’s no such thing as a free lunch. Yes, the sun sits at the center of the Solar System’s gravitational well, so in a certain way of viewing things, the sun is “downhill” of the rest of the planets. You might think it shouldn’t cost a lot to move anything closer to the sun. But the planets are already in motion, and to get them to change orbits, you first have to change their velocity.
There are many methods of moving objects from one orbit to another. For our calculations, we’ll take perhaps the most straightforward one: the Hohmann transfer. In our case, the transfer begins with a reduction in a planet’s speed, which causes it to fall toward the sun. But as it falls, it will go faster. If we don’t do anything about it, the planet will simply swing around the sun and fly back out, following a long elliptical orbit. That’s no good for us, so we have to give it another push to park it in the orbit we want.
I like to think of the Hohmann transfer as the orbital equivalent of sending a ball down a hill to a friend. First you have to kick the ball to get it moving. This requires energy. The ball will continue rolling, picking up speed as it goes. If your friend doesn’t do anything, the ball will roll right past them. Instead, they have to kick it again, requiring another burst of energy, to stop the ball at their feet.
We can estimate the relationship between a planet’s orbit and speed, and the energy needed to move from one orbit to another, with the vis-viva equation. The name is Latin for “living force” and is a relic from Medieval conceptions of energy and motion. But I guarantee that our future descendants will still use it to calculate their energy budgets for moving a planet around.
Going back to the Earth, we could never hope to capture all of the sun’s output with kilometer-thick Dyson panels. But we could if we were a bit closer. If we were to move our planet to a tenth of its current orbit (or 0.1 astronomical units), we could cover 0.04 percent of the sun—a hundred-fold boost in energy production. But the act of moving our planet will cost around 10 times as much energy as we needed to unbind it.
Thankfully, with the increased energy capture rate, our ROI time decreases to only 10,000 years, even with a panel energy efficiency of only 10 percent. We can then enjoy the additional captured energy for eons to come.
For Mercury, moving doesn’t really work in our favor. The increased energy cost of moving it to 0.1 AU increases our payback time to a few thousand years.
Moving Jupiter to the same orbit—or at least the rocky bits at its center; we can leave the hydrogen and helium to drift)—costs an enormous amount of energy, around 1034 Joules. But for our efforts, we could cover almost 20 percent of the sun. It would still take us over a million years to see a positive return on investment, but after that, it would be totally worth it.
For slimmer, meter-thick panels operating at 90 percent efficiency, the game totally changes. At 0.1 AU, the Earth would smear out a third of the sun, and we would get a return on our energy investment in around a year. As for Jupiter, we wouldn’t even have to go to 0.1 AU. At a distance about 30 percent further out than that, we could achieve the unimaginable: completely enclosing our sun. We would recoup our energy cost in only a few hundred years, and we could then possess the entirety of the sun’s output from then on.
So there you have it: Depending on our level of commitment and engineering ingenuity, we could follow Dyson’s recommendations and restructure our Solar System, capturing a significant fraction of the sun’s output and putting that energy towards whatever purpose we wish. But like I said, I don’t know how to actually go about achieving Dysonhood—I’ll leave that as a homework exercise for my engineering friends.